Chemical Reaction Engineering GATE-2005

Q 1: For the reaction 2R + S → T, the rates of formation, r_R, r_S, and rT of the substances R, S, and T respectively, are related by

A) 2r_R=r_S=r_T
B) 2r_R=r_S=-r_T
C) r_R=2r_S=2r_T
D) r_R=2r_S=-2r_T

Show Answer

Q 2: For the liquid phase reaction A → P, in a series of experiments in a batch reactor, the half-life t_1/2 was found to be inversely proportional to the square root of the initial concentration of A. The order of the reaction is

A) 3/2
B) 1
C) 1/2
D) -1/2

Show Answer

Q 3: Which is the correct statement from the following statements on the Arrhenius model of the rate constant k=Ae^{-E/RT} ?

A) A is always dimensionless
B) For two reactions 1 and 2, if A₁ = A₂ and E₁ > E₂, then k₁ (T) > k₂ (T)
C) For a given reaction, the % change of k with respect to temperature is higher at lower temperatures
D) The % change of k with respect to temperature is higher for higher A

Show Answer

Q 4: The rate expression for the reaction of A is given by

-r_A=\frac{k_1C_A^2}{1+k_2C_A^{1/2}}

The units of k₁ and k₂ are, respectively,

A) (mol^-1 m³ s^-1), (mol^-1/2 m^3/2)
B) (mol^-1 m³ s^-1), (mol^1/2 m^-3/2)
C) (mol m^-3 s^-1), (mol^1/2 m^-3/2 s^-1)
D) (mol^-1 m³ s^-1), (mol^-1/2 m^3/2 s^-1/2)

Show Answer

Q 5: The first-order liquid phase reaction A → P is to be carried out isothermally in the following ideal reactor configurations.

(P) A 1 m³ CSTR followed by a 1 m³ PFR.
(Q) A 2 m³ CSTR.
(R) A 1 m³ PFR followed by a 1 m³ CSTR.
(S) A 1 m³ CSTR followed by a 1 m³ CSTR.

The overall exit conversions, X, for the above configurations P, Q, R, and S, assuming identical inlet conditions and temperature, are related as

A) X_P > X_R > X_S > X_Q
B) X_P = X_R > X_S > X_Q
C) X_P = X_S = X_Q = X_R
D) X_Q > X_P > X_R > X_S

Show Answer

Q 6: The gas phase reaction A → B + C is carried out in an ideal PFR achieving a 40 % conversion of A. The feed has 70 mol% A and 30 mol% inerts. The inlet temperature is 300 K and the outlet temperature is 400 K. The ratio of the outlet to inlet molar concentration of A (assuming an ideal gas mixture and uniform pressure) is

A) 0.60
B) 0.30
C) 0.47
D) 0.35

Show Answer

Q 7: Match the items in Group I with those in Group II Group

Group I	Group II
P: Porous catalyst	1: Selectivity
Q: Parallel reaction	2: Shrinking core model
R: Non-ideal tubular reactor	3: Thiele modulus
S: Gas-solid non-catalytic reaction	4: Dispersion number

A) P-3, Q-1, R-4, S-2
B) P-1, Q-3, R-2, S-4
C) P-1, Q-4, R-2, S-3
D) P-3, Q-4, R-1, S-2

Show Answer

Q 8: The rate of the liquid phase reversible reaction A↔2B in (kmol m^-3 min^-1) at 298 K, is

-r_A=0.02C_A-0.01C_B

where the concentrations C_A and C_B are expressed in (kmol m^-3). What is the maximum limiting conversion of A achievable in an isothermal CSTR at 298 K, assuming pure A is fed at the inlet

A) 1
B) 2/3
C) 1/2
D) 1/3

Show Answer

Statement linked questions Q 9 & 10:

The residence time distribution E(t) (as shown below) of a reactor is zero until 3 minutes and then increases linearly to a maximum value E_max at 8 minutes after which it decreases linearly back to zero at 15 minutes.

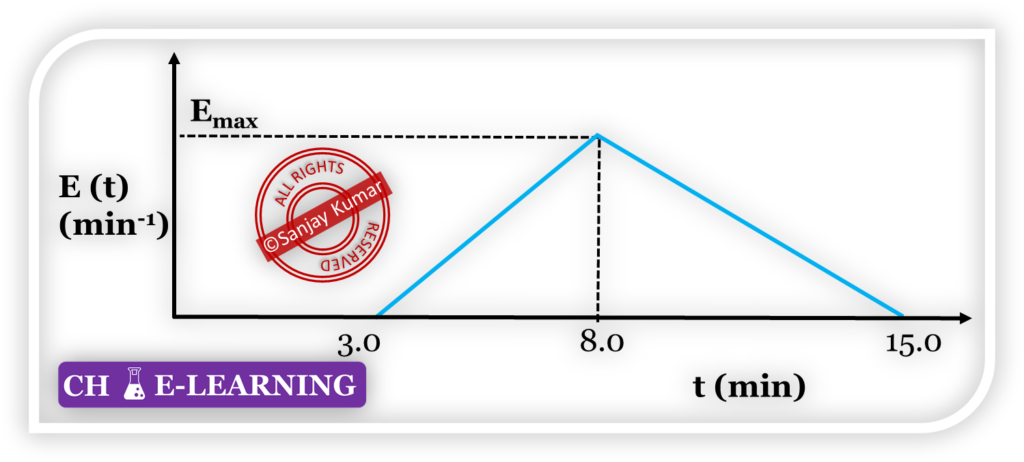
Q 9: What is the value of E_max?

A) 1/6
B) 1/8
C) 1/4
D) 1/3

Show Answer

Q 10: What is the value of the mean residence time in minutes?

A) 5.7
B) 8
C) 8.7
D) 12

Show Answer