Differentiate between ODE & PDE?4 min read

In this post, you will learn that what is the difference between ordinary differential equations (ODE) & partial differential equations (PDE).
First, we will start with dependent and independent variables.
Dependent & independent Variable:
Take any equation which gives the relationship between two variables i.e., x & y.
y=2x^2- Compute the value of y by taking x as 1, 2 & 3.
y=2\left(1\right)^2=2;\;\;y=2\left(2\right)^2=8;\;y=2\left(3\right)^2=18
- Here, the value of y depends on the value of x.
- So, we can say that variable x is independent while variable y is dependent.

- Independent variables can be more than one.

- These variables are important factors in any chemical process.
- Consider a plug flow reactor in which reactant A of concentration CA0 is reacting.

- The concentration of reactant A decreases along the length of PFR.
- It means the concentration is a function of spatial coordinate along the length of the reactor.
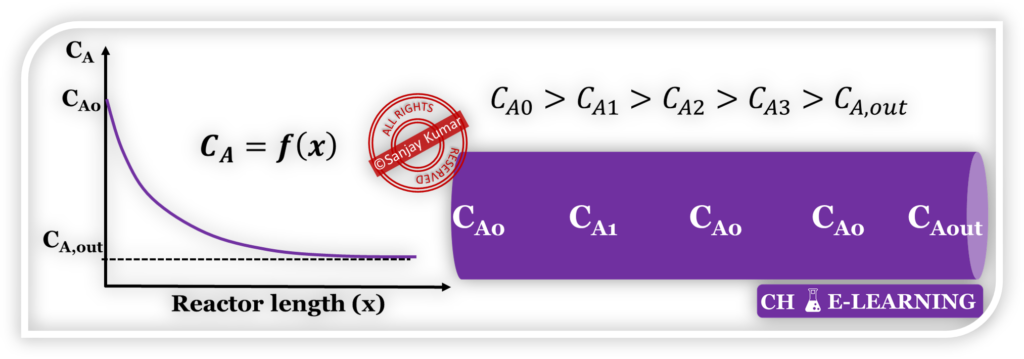
- In the above example, we are more interested to develop the relationship between concentration (CA) & spatial coordinate (x) and their rate change (dCA/dx).
- A differential equation is needed to observe how physical values like concentration, temperature, and so on vary with independent variables such as space and time.
Differential Equation:
These equations are used to express many general laws of nature.
- It provides a relationship between dependent and independent variables.
- Depending on the no. of independent variables, the differential equation can be categorized as Ordinary differential derivative (ODE) & Partial differential derivative (PDE).
ODE:
It contains only one independent variable. So, the derivative of the dependent variable with respect to the independent variable is the ordinary derivative.
\frac{dy}{dx}-ax=0;\;\;\;\;\frac{d^3y}{{dx}^3}+\frac yx-b=0Rate equation,
-\frac{dC_A}{dt}=k\left(C_A\right)^n- It involves differentials with respect to only one independent variable.
- For example, in the above equation, the independent variable is x and the solution is y(x).
PDE:
It contains several independent variables. So, the derivative of the dependent variable with respect to one independent variable while holding other independent variables constant is a partial derivative.
\frac{\partial z}{\partial x}-\frac{\partial z}{\partial y}=0;\;\;\;\;\frac{\partial^2z}{\partial x\partial y}+\frac{\partial z}{\partial x}+z=0Heat Conduction equation,
\frac{\partial^2T}{\partial^2x}+\frac{\partial^2T}{\partial^2y}+\frac{\partial^2T}{\partial^2z}=\frac1\alpha\frac{\partial T}{\partial t}Fick’s 2nd law equation,
\frac{\partial^2C}{\partial^2x}+\frac{\partial^2C}{\partial^2y}+\frac{\partial^2C}{\partial^2z}=\frac1D\frac{\partial T}{\partial t}- It involves differentials with respect to many independent variables.
- For example, in the above equation, the independent variables are x, y, and the solution is y(x, y).
- PDEs have a more complex solution than ODEs.

Its like you read my mind You appear to know so much about this like you wrote the book in it or something I think that you can do with a few pics to drive the message home a little bit but instead of that this is excellent blog A fantastic read Ill certainly be back